Cherak Z, Loucif L, Moussi A, Rolain J-M.
Epidemiology of mobile colistin resistance (mcr) genes in aquatic environments. Journal of Global Antimicrobial Resistance [Internet]. 2021;27 :51-62.
Publisher's VersionAbstract
Colistin is one of the last-line therapies against multidrug-resistant Gram-negative pathogens, especially carbapenemase-producing isolates, making resistance to this compound a major global public-health crisis. Until recently, colistin resistance in Gram-negative bacteria was known to arise only by chromosomal mutations. However, a plasmid-mediated colistin resistance mechanism was described in late 2015. This mechanism is encoded by different mobile colistin resistance (mcr) genes that encode phosphoethanolamine (pEtN) transferases. These enzymes catalyse the addition of a pEtN moiety to lipid A in the bacterial outer membrane leading to colistin resistance. MCR-producing Gram-negative bacteria have been largely disseminated worldwide. However, their environmental dissemination has been underestimated. Indeed, water environments act as a connecting medium between different environments, allowing them to play a crucial role in the spread of antibiotic resistance between the natural environment and humans and other animals. For a better understanding of the role of such environments as reservoirs and/or dissemination routes of mcr genes, this review discusses primarily the various water habitats contributing to the spread of antibiotic resistance. Thereafter, we provide an overview of existing knowledge regarding the global epidemiology of mcr genes in water environments. This review confirms the global distribution of mcr genes in several water environments, including wastewater from different origins, surface water and tap water, making these environments reservoirs and dissemination routes of concern for this resistance mechanism.
BOUAFIA W.
Evaluation des activités biologiques et caractérisation phytochimique de la plante Ephedra altissima Desf. Biologie des organismes [Internet]. 2021.
Publisher's VersionAbstract
Ephedra altissima Desf. est une plante médicinale de la famille des Ephedraceae, largement utilisée en médecine traditionnelle pour le traitement des maladies respiratoires et le diabète. L’objectif de la présente étude est l’évaluation des activités biologiques in vitro et la caractérisation phytochimique des extraits (éther de pétrole, acétate d’éthyle et n-butanol) obtenus à partir de la plante Ephedra altissima. Des dosages colorimétriques ont été effectués pour révéler la présence de différentes classes de métabolites secondaires. La détermination des teneurs en polyphénols, flavonoïdes et tanins condensés a été réalisée par des dosages spectrophotométriques. L’activité antioxydante a été évaluée par huit méthodes différentes, l’activité anti-artéritiques a été réalisée par la méthode d’inhibition de la dénaturation du sérum d’albumine bovin, les activités inhibitrices de l’α-amylase et de la lipase pancréatique in vitro ont été estimées par des méthodes colorimétriques en utilisant de l'amidon et du p-nitrophényl butyrate en tant que substrats. Les conditions physiologiques de la digestion humaine ont été simulées par le modèle de la digestion gastro-intestinale (DGI) in vitro. L’activité antibactérienne a été déterminée par la méthode de diffusion sur disques en milieu gélosé contre sept souches bacteriennes. L’analyse et la quantification des composés phénoliques ont été effectuées par LC-DAD-ESI/MS. L’investigation phytochimique de l’extrait n-butanolique a été réalisée par l’utilisation en alternance de diverses méthodes chromatographiques (VLC, CC, CCE et CCM). Les structures des deux composés isolés A et B ont été identifiées par analyse spectroscopique ESI-MS, l’UV et la RMN dans ses multiples techniques, RMN 1H, RMN 13C, et RMN 2D (COSY, HSQC, HMBC et TOCSY). A l’issue de ces travaux, nous retiendrons la richesse de cette plante en métabolites secondaires. En effet, l’extrait AcOEt présente la teneur la plus élevée en polyphénols (125,62 μg EAG mg-1), et le n-BuOH possède la teneur la plus élevée en flavonoïdes (19,18 μg EQ mg-1). Tous les extraits ont des activités antioxydantes dans divers systèmes et l'extrait aqueux a montré une bonne activité antioxydante par la méthode potentiométrique. L’extrait AcOEt exhibe la plus forte activité anti-artéritique, activités inhibitrices de de l’α-amylase et de la lipase pancréatique avec des valeurs de CI50 (126,4 ± 2,36, 8,07 ± 0,15 et 289,1 ± 0,53 μg/ml) respectivement. Tous les extraits testés exercent une activité antibactérienne dose dépendante contre au moins trois souches avec des CMI allant de (3,12 à 50 μg/ml). L’analyse par LC-DAD-ESI/MS a permis de détecter la présence de dix-neuf composés différents, huit sont des acides phénoliques simples et des dérivés d'acide phénolique, et onze sont des flavonoïdes liés à des groupes glycosyles. L’investigation phytochimique réalisée sur l’extrait n-batanolique conduit à l’isolement et identification structurale de deux flavonoides glycosylés nommés : Isorhamnétine 3-O- α-L-rhamnopyranosyl (1’’’-6’’)- β-D-galactopyranoside et Kaempférol 3-O- α-L-rhamnopyranosyl (1’’’-6’’)- β-D-glucopyranoside.
Alloui N, Sellaoui S, Ayachi A, Bennoune O.
Evaluation of biosecurity practices in a laying hens farm using Biocheck. UGent. Multidisciplinary Science Journal [Internet]. 2021;3 (3) :e2021014-e2021014.
Publisher's VersionAbstract
The Biocheck.UGent scoring system was developed to measure and quantify the level of biosecurity in animal husbandry. This tool is composed of all the relevant elements of biosecurity in poultry farms (broilers and laying hens) and is subdivided into external and internal biosecurity. The peculiarity of this scoring system is that it takes into account the relative importance of the different aspects of biosecurity, resulting in a risk-weighted score. The biosecurity scores obtained are provided immediately after completing the questionnaire and the scores for each sub-category can be compared to global averages to allow the poultry farmer to compare the results obtained and correct any anomalies that are on their farm. In Algeria, preliminary results from a survey in poultry houses of 30.000 laying hens show a wide range of biosecurity levels on that farm, with internal biosecurity scores ranging from 6 to 72% and external biosecurity scores ranging from 28 to 92% in the subgroups. The overall scores were 50% and 54%, respectively. These early results show that despite the well-known importance of biosecurity, there is a lack of implementation of many biosecurity measures.
Alloui N, Sellaoui S, Ayachi A, Bennoune O.
Evaluation of biosecurity practices in a laying hens farm using Biocheck. UGent. Multidisciplinary Science Journal [Internet]. 2021;3 (3) :e2021014-e2021014.
Publisher's VersionAbstract
The Biocheck.UGent scoring system was developed to measure and quantify the level of biosecurity in animal husbandry. This tool is composed of all the relevant elements of biosecurity in poultry farms (broilers and laying hens) and is subdivided into external and internal biosecurity. The peculiarity of this scoring system is that it takes into account the relative importance of the different aspects of biosecurity, resulting in a risk-weighted score. The biosecurity scores obtained are provided immediately after completing the questionnaire and the scores for each sub-category can be compared to global averages to allow the poultry farmer to compare the results obtained and correct any anomalies that are on their farm. In Algeria, preliminary results from a survey in poultry houses of 30.000 laying hens show a wide range of biosecurity levels on that farm, with internal biosecurity scores ranging from 6 to 72% and external biosecurity scores ranging from 28 to 92% in the subgroups. The overall scores were 50% and 54%, respectively. These early results show that despite the well-known importance of biosecurity, there is a lack of implementation of many biosecurity measures.
Alloui N, Sellaoui S, Ayachi A, Bennoune O.
Evaluation of biosecurity practices in a laying hens farm using Biocheck. UGent. Multidisciplinary Science Journal [Internet]. 2021;3 (3) :e2021014-e2021014.
Publisher's VersionAbstract
The Biocheck.UGent scoring system was developed to measure and quantify the level of biosecurity in animal husbandry. This tool is composed of all the relevant elements of biosecurity in poultry farms (broilers and laying hens) and is subdivided into external and internal biosecurity. The peculiarity of this scoring system is that it takes into account the relative importance of the different aspects of biosecurity, resulting in a risk-weighted score. The biosecurity scores obtained are provided immediately after completing the questionnaire and the scores for each sub-category can be compared to global averages to allow the poultry farmer to compare the results obtained and correct any anomalies that are on their farm. In Algeria, preliminary results from a survey in poultry houses of 30.000 laying hens show a wide range of biosecurity levels on that farm, with internal biosecurity scores ranging from 6 to 72% and external biosecurity scores ranging from 28 to 92% in the subgroups. The overall scores were 50% and 54%, respectively. These early results show that despite the well-known importance of biosecurity, there is a lack of implementation of many biosecurity measures.
Alloui N, Sellaoui S, Ayachi A, Bennoune O.
Evaluation of biosecurity practices in a laying hens farm using Biocheck. UGent. Multidisciplinary Science Journal [Internet]. 2021;3 (3) :e2021014-e2021014.
Publisher's VersionAbstract
The Biocheck.UGent scoring system was developed to measure and quantify the level of biosecurity in animal husbandry. This tool is composed of all the relevant elements of biosecurity in poultry farms (broilers and laying hens) and is subdivided into external and internal biosecurity. The peculiarity of this scoring system is that it takes into account the relative importance of the different aspects of biosecurity, resulting in a risk-weighted score. The biosecurity scores obtained are provided immediately after completing the questionnaire and the scores for each sub-category can be compared to global averages to allow the poultry farmer to compare the results obtained and correct any anomalies that are on their farm. In Algeria, preliminary results from a survey in poultry houses of 30.000 laying hens show a wide range of biosecurity levels on that farm, with internal biosecurity scores ranging from 6 to 72% and external biosecurity scores ranging from 28 to 92% in the subgroups. The overall scores were 50% and 54%, respectively. These early results show that despite the well-known importance of biosecurity, there is a lack of implementation of many biosecurity measures.
Mohamadi A, Demdoum A, Bouaicha F, MENANI MR.
Evaluation of the quality of groundwater for its appropriateness for irrigation purposes using Water Quality Index (WQI), Mchira-Teleghma aquifer case study, northeastern Algeria. Sustainable Water Resources Management [Internet]. 2021;7 :1-16.
Publisher's VersionAbstract
The Mio-Plio-Quaternary groundwater of Mchira-Teleghma suffers from an increasing rate of salinity especially in the northwestern part. To identify the reason for the water’s salinity and its aptitude for irrigation, physico-chemical analyses of 20 water samples, which were based on the different physical and chemical parameters (electric conductivity EC, pH, Ca2+, Mg2+, Na+, K+, HCO3−, Cl−, SO42−, NO3− and Sr2+), were carried out during the period of October 2015. This study showed disquieting anomalies of electric conductivity that reached the value of 4376.14 µS cm−1. The statistical analyses, the multivariate statistics: the principal component analysis, Q-mode cluster analyses, Sr2+/Ca2+ ratio and water type showed that the hydrochemistry of Mchira-Teleghma groundwater is controlled by the dissolution of carbonate rocks and the leaching of evaporite processes, which proved that these anomalies of the MPQ groundwater’s salinity of Mchira-Teleghma are mainly determined by the leaching of Triassic gypsum formations process. This hydrogeochemical process generates an unsuitable quality of water based on Wilcox’s and Water Quality Index’s methods, whereas Richard’s method classifies all water samples to C3S1 and C4S1 classes as they are recommended to be used with salt-tolerant species in well-drained and leached soils.
Mohamadi A, Demdoum A, Bouaicha F, MENANI MR.
Evaluation of the quality of groundwater for its appropriateness for irrigation purposes using Water Quality Index (WQI), Mchira-Teleghma aquifer case study, northeastern Algeria. Sustainable Water Resources Management [Internet]. 2021;7 :1-16.
Publisher's VersionAbstract
The Mio-Plio-Quaternary groundwater of Mchira-Teleghma suffers from an increasing rate of salinity especially in the northwestern part. To identify the reason for the water’s salinity and its aptitude for irrigation, physico-chemical analyses of 20 water samples, which were based on the different physical and chemical parameters (electric conductivity EC, pH, Ca2+, Mg2+, Na+, K+, HCO3−, Cl−, SO42−, NO3− and Sr2+), were carried out during the period of October 2015. This study showed disquieting anomalies of electric conductivity that reached the value of 4376.14 µS cm−1. The statistical analyses, the multivariate statistics: the principal component analysis, Q-mode cluster analyses, Sr2+/Ca2+ ratio and water type showed that the hydrochemistry of Mchira-Teleghma groundwater is controlled by the dissolution of carbonate rocks and the leaching of evaporite processes, which proved that these anomalies of the MPQ groundwater’s salinity of Mchira-Teleghma are mainly determined by the leaching of Triassic gypsum formations process. This hydrogeochemical process generates an unsuitable quality of water based on Wilcox’s and Water Quality Index’s methods, whereas Richard’s method classifies all water samples to C3S1 and C4S1 classes as they are recommended to be used with salt-tolerant species in well-drained and leached soils.
Mohamadi A, Demdoum A, Bouaicha F, MENANI MR.
Evaluation of the quality of groundwater for its appropriateness for irrigation purposes using Water Quality Index (WQI), Mchira-Teleghma aquifer case study, northeastern Algeria. Sustainable Water Resources Management [Internet]. 2021;7 :1-16.
Publisher's VersionAbstract
The Mio-Plio-Quaternary groundwater of Mchira-Teleghma suffers from an increasing rate of salinity especially in the northwestern part. To identify the reason for the water’s salinity and its aptitude for irrigation, physico-chemical analyses of 20 water samples, which were based on the different physical and chemical parameters (electric conductivity EC, pH, Ca2+, Mg2+, Na+, K+, HCO3−, Cl−, SO42−, NO3− and Sr2+), were carried out during the period of October 2015. This study showed disquieting anomalies of electric conductivity that reached the value of 4376.14 µS cm−1. The statistical analyses, the multivariate statistics: the principal component analysis, Q-mode cluster analyses, Sr2+/Ca2+ ratio and water type showed that the hydrochemistry of Mchira-Teleghma groundwater is controlled by the dissolution of carbonate rocks and the leaching of evaporite processes, which proved that these anomalies of the MPQ groundwater’s salinity of Mchira-Teleghma are mainly determined by the leaching of Triassic gypsum formations process. This hydrogeochemical process generates an unsuitable quality of water based on Wilcox’s and Water Quality Index’s methods, whereas Richard’s method classifies all water samples to C3S1 and C4S1 classes as they are recommended to be used with salt-tolerant species in well-drained and leached soils.
Mohamadi A, Demdoum A, Bouaicha F, MENANI MR.
Evaluation of the quality of groundwater for its appropriateness for irrigation purposes using Water Quality Index (WQI), Mchira-Teleghma aquifer case study, northeastern Algeria. Sustainable Water Resources Management [Internet]. 2021;7 :1-16.
Publisher's VersionAbstract
The Mio-Plio-Quaternary groundwater of Mchira-Teleghma suffers from an increasing rate of salinity especially in the northwestern part. To identify the reason for the water’s salinity and its aptitude for irrigation, physico-chemical analyses of 20 water samples, which were based on the different physical and chemical parameters (electric conductivity EC, pH, Ca2+, Mg2+, Na+, K+, HCO3−, Cl−, SO42−, NO3− and Sr2+), were carried out during the period of October 2015. This study showed disquieting anomalies of electric conductivity that reached the value of 4376.14 µS cm−1. The statistical analyses, the multivariate statistics: the principal component analysis, Q-mode cluster analyses, Sr2+/Ca2+ ratio and water type showed that the hydrochemistry of Mchira-Teleghma groundwater is controlled by the dissolution of carbonate rocks and the leaching of evaporite processes, which proved that these anomalies of the MPQ groundwater’s salinity of Mchira-Teleghma are mainly determined by the leaching of Triassic gypsum formations process. This hydrogeochemical process generates an unsuitable quality of water based on Wilcox’s and Water Quality Index’s methods, whereas Richard’s method classifies all water samples to C3S1 and C4S1 classes as they are recommended to be used with salt-tolerant species in well-drained and leached soils.
Bensaad M-S, Dassamiour S, Hambaba L, Kahoul M-A, Benhoula M.
Evidence of anti-inflammatory and anti-ulcer properties of aerial parts of Centaurea tougourensis Boiss. and Reut. Tropical Journal of Pharmaceutical Research [Internet]. 2021;20 (8).
Publisher's VersionAbstract
Purpose: To determine the anti-inflammatory and anti-ulcer properties of the aerial parts of Centaurea tougourensis Boiss. & Reut.
Methods: The effects of n-butanol (n-BuOH) extract of the aerial part of Centaurea tougourensis on carrageenan-induced paw edema and ethanol-induced gastric mucosal damage were determined at 2 doses (200 and 400 mg/kg, po) in a mouse model. For each test, the animals were randomly divided into negative and positive control groups, as well as extract-treated groups. The mice were observed for any sign of inflammation for a period of 24h.
Results: Reduction of paw edema by C. tougourensis extract was highly significant (p < 0.001) at a dose of 400 mg/kg 24 h after carrageenan injection, with 55.26 % inhibition, followed very closely by 53.15 % inhibition at the dose of 200 mg/kg; indomethacin group showed an inhibition of 60 %. Histological examination supported the inhibition results. A significant reduction in inflammation by the extract at a dose of 400 mg/kg was also observed. No sign of ulcer was observed with C. tougourensis at the two doses (200 and 400 mg/kg). The total polyphenol content of the n-BuOH extract was 85.44 цg gallic acid equivalent/mg of extract. Tannins were the most abundant fraction (51.87 цg tannic acid equivalent/mg of extract), followed by flavonoids (25.55 цg quercetin equivalent/mg of extract).
Conclusion: The results indicate that C. tougourensis may have potential beneficial effects in the treatment of diseases associated with inflammation and pain, besides its protective effect on the gastrointestinal tract.
Bensaad M-S, Dassamiour S, Hambaba L, Kahoul M-A, Benhoula M.
Evidence of anti-inflammatory and anti-ulcer properties of aerial parts of Centaurea tougourensis Boiss. and Reut. Tropical Journal of Pharmaceutical Research [Internet]. 2021;20 (8).
Publisher's VersionAbstract
Purpose: To determine the anti-inflammatory and anti-ulcer properties of the aerial parts of Centaurea tougourensis Boiss. & Reut.
Methods: The effects of n-butanol (n-BuOH) extract of the aerial part of Centaurea tougourensis on carrageenan-induced paw edema and ethanol-induced gastric mucosal damage were determined at 2 doses (200 and 400 mg/kg, po) in a mouse model. For each test, the animals were randomly divided into negative and positive control groups, as well as extract-treated groups. The mice were observed for any sign of inflammation for a period of 24h.
Results: Reduction of paw edema by C. tougourensis extract was highly significant (p < 0.001) at a dose of 400 mg/kg 24 h after carrageenan injection, with 55.26 % inhibition, followed very closely by 53.15 % inhibition at the dose of 200 mg/kg; indomethacin group showed an inhibition of 60 %. Histological examination supported the inhibition results. A significant reduction in inflammation by the extract at a dose of 400 mg/kg was also observed. No sign of ulcer was observed with C. tougourensis at the two doses (200 and 400 mg/kg). The total polyphenol content of the n-BuOH extract was 85.44 цg gallic acid equivalent/mg of extract. Tannins were the most abundant fraction (51.87 цg tannic acid equivalent/mg of extract), followed by flavonoids (25.55 цg quercetin equivalent/mg of extract).
Conclusion: The results indicate that C. tougourensis may have potential beneficial effects in the treatment of diseases associated with inflammation and pain, besides its protective effect on the gastrointestinal tract.
Bensaad M-S, Dassamiour S, Hambaba L, Kahoul M-A, Benhoula M.
Evidence of anti-inflammatory and anti-ulcer properties of aerial parts of Centaurea tougourensis Boiss. and Reut. Tropical Journal of Pharmaceutical Research [Internet]. 2021;20 (8).
Publisher's VersionAbstract
Purpose: To determine the anti-inflammatory and anti-ulcer properties of the aerial parts of Centaurea tougourensis Boiss. & Reut.
Methods: The effects of n-butanol (n-BuOH) extract of the aerial part of Centaurea tougourensis on carrageenan-induced paw edema and ethanol-induced gastric mucosal damage were determined at 2 doses (200 and 400 mg/kg, po) in a mouse model. For each test, the animals were randomly divided into negative and positive control groups, as well as extract-treated groups. The mice were observed for any sign of inflammation for a period of 24h.
Results: Reduction of paw edema by C. tougourensis extract was highly significant (p < 0.001) at a dose of 400 mg/kg 24 h after carrageenan injection, with 55.26 % inhibition, followed very closely by 53.15 % inhibition at the dose of 200 mg/kg; indomethacin group showed an inhibition of 60 %. Histological examination supported the inhibition results. A significant reduction in inflammation by the extract at a dose of 400 mg/kg was also observed. No sign of ulcer was observed with C. tougourensis at the two doses (200 and 400 mg/kg). The total polyphenol content of the n-BuOH extract was 85.44 цg gallic acid equivalent/mg of extract. Tannins were the most abundant fraction (51.87 цg tannic acid equivalent/mg of extract), followed by flavonoids (25.55 цg quercetin equivalent/mg of extract).
Conclusion: The results indicate that C. tougourensis may have potential beneficial effects in the treatment of diseases associated with inflammation and pain, besides its protective effect on the gastrointestinal tract.
Bensaad M-S, Dassamiour S, Hambaba L, Kahoul M-A, Benhoula M.
Evidence of anti-inflammatory and anti-ulcer properties of aerial parts of Centaurea tougourensis Boiss. and Reut. Tropical Journal of Pharmaceutical Research [Internet]. 2021;20 (8).
Publisher's VersionAbstract
Purpose: To determine the anti-inflammatory and anti-ulcer properties of the aerial parts of Centaurea tougourensis Boiss. & Reut.
Methods: The effects of n-butanol (n-BuOH) extract of the aerial part of Centaurea tougourensis on carrageenan-induced paw edema and ethanol-induced gastric mucosal damage were determined at 2 doses (200 and 400 mg/kg, po) in a mouse model. For each test, the animals were randomly divided into negative and positive control groups, as well as extract-treated groups. The mice were observed for any sign of inflammation for a period of 24h.
Results: Reduction of paw edema by C. tougourensis extract was highly significant (p < 0.001) at a dose of 400 mg/kg 24 h after carrageenan injection, with 55.26 % inhibition, followed very closely by 53.15 % inhibition at the dose of 200 mg/kg; indomethacin group showed an inhibition of 60 %. Histological examination supported the inhibition results. A significant reduction in inflammation by the extract at a dose of 400 mg/kg was also observed. No sign of ulcer was observed with C. tougourensis at the two doses (200 and 400 mg/kg). The total polyphenol content of the n-BuOH extract was 85.44 цg gallic acid equivalent/mg of extract. Tannins were the most abundant fraction (51.87 цg tannic acid equivalent/mg of extract), followed by flavonoids (25.55 цg quercetin equivalent/mg of extract).
Conclusion: The results indicate that C. tougourensis may have potential beneficial effects in the treatment of diseases associated with inflammation and pain, besides its protective effect on the gastrointestinal tract.
Bensaad M-S, Dassamiour S, Hambaba L, Kahoul M-A, Benhoula M.
Evidence of anti-inflammatory and anti-ulcer properties of aerial parts of Centaurea tougourensis Boiss. and Reut. Tropical Journal of Pharmaceutical Research [Internet]. 2021;20 (8).
Publisher's VersionAbstract
Purpose: To determine the anti-inflammatory and anti-ulcer properties of the aerial parts of Centaurea tougourensis Boiss. & Reut.
Methods: The effects of n-butanol (n-BuOH) extract of the aerial part of Centaurea tougourensis on carrageenan-induced paw edema and ethanol-induced gastric mucosal damage were determined at 2 doses (200 and 400 mg/kg, po) in a mouse model. For each test, the animals were randomly divided into negative and positive control groups, as well as extract-treated groups. The mice were observed for any sign of inflammation for a period of 24h.
Results: Reduction of paw edema by C. tougourensis extract was highly significant (p < 0.001) at a dose of 400 mg/kg 24 h after carrageenan injection, with 55.26 % inhibition, followed very closely by 53.15 % inhibition at the dose of 200 mg/kg; indomethacin group showed an inhibition of 60 %. Histological examination supported the inhibition results. A significant reduction in inflammation by the extract at a dose of 400 mg/kg was also observed. No sign of ulcer was observed with C. tougourensis at the two doses (200 and 400 mg/kg). The total polyphenol content of the n-BuOH extract was 85.44 цg gallic acid equivalent/mg of extract. Tannins were the most abundant fraction (51.87 цg tannic acid equivalent/mg of extract), followed by flavonoids (25.55 цg quercetin equivalent/mg of extract).
Conclusion: The results indicate that C. tougourensis may have potential beneficial effects in the treatment of diseases associated with inflammation and pain, besides its protective effect on the gastrointestinal tract.
Messaoudi SA, Bouhoufani O, Hamchi I, Alahyane M.
Existence and blow up in a system of wave equations with nonstandard nonlinearities. Electronic Journal of Differential Equations [Internet]. 2021;91 :1–33.
Publisher's VersionAbstract
In this article, we consider a coupled system of two nonlinear hyperbolic equations, where the exponents in the damping and source terms are variables. First, we prove a theorem of existence and uniqueness of weak solution, by using the Faedo Galerkin approximations and the Banach fixed point theorem. Then, using the energy method, we show that certain solutions with positive initial energy blow up in finite time. We also give some numerical applications to illustrate our theoretical results.
Messaoudi SA, Bouhoufani O, Hamchi I, Alahyane M.
Existence and blow up in a system of wave equations with nonstandard nonlinearities. Electronic Journal of Differential Equations [Internet]. 2021;91 :1–33.
Publisher's VersionAbstract
In this article, we consider a coupled system of two nonlinear hyperbolic equations, where the exponents in the damping and source terms are variables. First, we prove a theorem of existence and uniqueness of weak solution, by using the Faedo Galerkin approximations and the Banach fixed point theorem. Then, using the energy method, we show that certain solutions with positive initial energy blow up in finite time. We also give some numerical applications to illustrate our theoretical results.
Messaoudi SA, Bouhoufani O, Hamchi I, Alahyane M.
Existence and blow up in a system of wave equations with nonstandard nonlinearities. Electronic Journal of Differential Equations [Internet]. 2021;91 :1–33.
Publisher's VersionAbstract
In this article, we consider a coupled system of two nonlinear hyperbolic equations, where the exponents in the damping and source terms are variables. First, we prove a theorem of existence and uniqueness of weak solution, by using the Faedo Galerkin approximations and the Banach fixed point theorem. Then, using the energy method, we show that certain solutions with positive initial energy blow up in finite time. We also give some numerical applications to illustrate our theoretical results.
Messaoudi SA, Bouhoufani O, Hamchi I, Alahyane M.
Existence and blow up in a system of wave equations with nonstandard nonlinearities. Electronic Journal of Differential Equations [Internet]. 2021;91 :1–33.
Publisher's VersionAbstract
In this article, we consider a coupled system of two nonlinear hyperbolic equations, where the exponents in the damping and source terms are variables. First, we prove a theorem of existence and uniqueness of weak solution, by using the Faedo Galerkin approximations and the Banach fixed point theorem. Then, using the energy method, we show that certain solutions with positive initial energy blow up in finite time. We also give some numerical applications to illustrate our theoretical results.
BOUGOFFA LAZHAR, Rach RC, MENNOUNI ABDELAZIZ.
On the existence, uniqueness, and new analytic approximate solution of the modified error function in two‐phase Stefan problems. Mathematical Methods in the Applied Sciences [Internet]. 2021;44 :10948-10956.
Publisher's VersionAbstract
This paper provides a new proof of the existence and uniqueness of the solution for a nonlinear boundary value problem
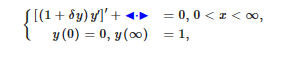
which describes the study of two-phase Stefan problems on the semi-infinite line [0, ∞). This result considerably extends the analysis of a recent work. A highly accurate analytic approximate solution of this problem is also provided via the Adomian decomposition method.